

Custom Search
|
|

|
||
 CONCEPTS OF METERING FLUID FLOW
CHAPTER 3.
1. INTRODUCTION. Selection of the proper meter for measuring a flowing fluid
(gas, steam, or liquid) is often a complicated process. Knowledge of certain
fluid flow fundamentals will help in understanding the operating principles of
various meters and assist in meter selection.
2. COMPRESSIBLE AND INCOMPRESSIBLE FLOWS. Flows in which variations in
density are negligible are termed incompressible. Density of an
incompressible fluid is not affected by changes in pressure and velocity.
Flow rates of a compressible fluid are significantly affected by pressure and
temperature changes. These characteristics must be considered when measuring
the flow of fluids.
3. MEASURING FLUID FLOW. With most fluid flow measurement instruments, the
flow rate is determined inferentially by measuring the fluid's velocity or
change in kinetic energy. Velocity depends on the pressure differential that
is forcing the fluid through the pipe or conduit. Because the pipe's
cross-sectional area is constant, the average velocity is an indication of
flow rate. The basic relationship for determining the fluid's flow rate in
such cases is:
Q=VxA
where:
Q = flow rate
V = average fluid velocity
A = cross-sectional area of pipe
The equation can be adjusted to determine volumetric, mass, and heat flow
rates. For compressible fluids, pressure and/or temperature must be measured
to determine the fluid density. This changing density must be used in the
basic formula to determine a compensated flow rate.
3.1 Direct Measurement. Direct measurement of fluid flows are made using
positive displacement meters. These units cannot meter steam or other high
temperature gases. The flowing fluid is divided into specific measurable
units and totalized using mechanical or electronic counters.
3.2 Indirect Measurement. Differential pressure and velocity flowmeters
indirectly measure fluid flow rates. Many meters in use today operate on the
differential pressure concept. Such meters use the correlation between
pressure and velocity to determine the rate of flow. This correlation is
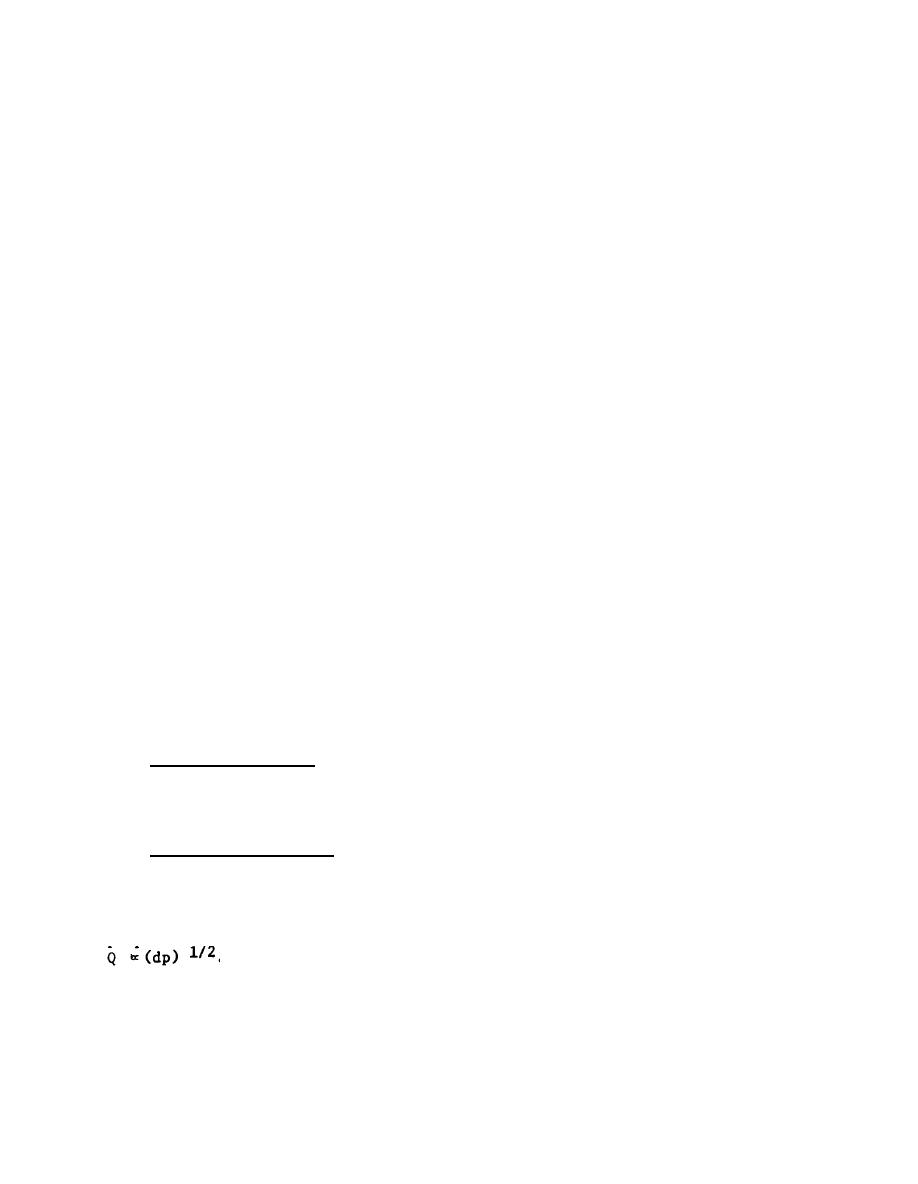
expressed by the differential pressure formula which states that flow is
proportional to the square root of the differential pressure, i.e.,
For velocity meters the relationship of the continuity
equation, Q = V x A, is applied. Velocity meters measure velocity at a point
or full bore and calculate flow. A correction factor must be applied to these
meters which results in accuracies of 95 to 99 percent.
3-1
|
 
|
|
 |
||